
control charts (X-bar and R-bar chart) on the selected parameters and see how it affects the quality production of Cement Company of Northern Nigeria Plc, Sokoto
1.0 CHAPTER ONE
GENERAL INTRODUCTION
1.1 INTRODUCTION
Control charts, also known as Shewhart charts or process behavior charts, in statistical process control are tools used to manufacturing or business process in a state on statistical control. When analysis of control chart indicates that the process is currently under (i.e is stable, with variation only coming from sources common to the process) then data from the process can be used to predict the future performance of the process. If the chart indicates that the process being monitored is not in control, analysis of the chart can help determined the source of variation, which can then be eliminated to bring the process back into control.
Control chart is a specific kind of run chart that allows significant change to be seen as part of an objective and disciplined approach that enables correct decisions regarding control of the process, including whether or not to change process control parameters. Process parameters should be adjusted for a process that is in control, as this will result in degraded process performance.
The control chart is one of the seven basis tools of quality control. The word 'quality' has different connotations when used by different people; the quality of products is satisfactory when the products are able to satisfy the needs of the consumers. The production of high quality involves many intermediate steps. The design engineer must be able to translate the stated needs of the consumers in to engineering, including specifications tolerances.
The product engineer must design a production process that will be able to produce a product meeting these satisfactions and tolerance. The production manager has the responsibility of actually producing the products. The quality inspector must test and evaluate the products been produced after the product is actually produced, the customer can identify exactly the modifications needed to make it perfectly satisfactory.
Quality control is a name to the collection of management’s techniques and devices to manage monitor and control all these steps in the production of a products of desired quality. It is more than a set of statistical formulas and tables for the acceptance and control to an enlightened management, it is must represent a business investment as many other investment should show a proper return to justify its existence. The quality control the responsibility of everyone in the company whatever work is performed by a person or machine, the person doing the work or operating the machines is the one who must effectively and efficiently control quality or make information available that the quality desired cannot be attained so that remedial can be taken. The application of statistic was made in the areas of astronomy, physics, biology and science, but it was until 1920's the statistical theory begun to be applied effectively to quality control. A factor in the birth of the statistical quality control in 20th century was developed, in immediately preceding years of an exact theory of sampling first to apply new statistical methods to the problems of quality control was Walter A Shewhart made the first sketch of modern control chart, another two men from Bell system H.F Dodge and H.G Roaming took the leadership in developing the application of statistical theory of sampling inspection (Gharret et al 1987). The work of Shewhart, Dodge and Roaming (1947) constitute much of what today's comprises the theory of statistical quality control, thus quality control help in determining the quality standard during the production process itself and saves the manufacturer of loses which he might have incurred due to non-maintenance of a predetermined quality level. The producer is basically interested in producing such quality items which would be readily acceptable to the prospective buyer or which would conform to certain quality levels about which both the producer and consumer are certain.
You May Also Like
- ANALYSIS OF SSCE EXAMINATION RESULT USING CANONICAL CORRELATION AND PRINCIPAL COMPONENT ANALYSES
- VOLATILITY MODELING OF DAILY EXCHANGE RATE BETWEEN UNITED STATES DOLLAR AND NIGERIA NAIRA
- Effect on population structure of Human Fertility
- Understanding the application of multiple linear regression model in health care research pdf
1.2 BASIC CONCEPT AND DEFINITIONS:
QUALITY: Quality is define as the term covering any and every characteristics, property or performance of a product or services that meet specific requirements. It is also defined as features that meet the needs for consumer and provide products satisfaction.
CONTROL: Is the manipulation condition of the process being about a desired change in the output characteristic of process. It is feedback loop through which we measure actual performance and compare it with standard of difference.
PROCESS: Is a sequence of operation by which such as labor, materials and methods are transferred into output in form of product or services.
CHART: A chart is defined as a pictorial representation of a data.
QUALITY CONTROL: Quality control can be defined as application of statistical method collection and analyzing instruction of data for setting the economic standard quality and maintaining adherence to standards so that the variation in products quality may be controlled.
VARIATION: Is a law in nature that no two items any categories are the same the variation is large and noticeable such as height of human being and variation may be small as weight and length of chalk.
COMMON CAUSE OF VARIATION: These courses are due to factors that are inherent in the design of the system and reflect the usual amount of variation to be expected.
ASSIGNABLE COURSE OF VARIATION: These courses are due to factors that are not part of the process design and not ordinarily part of the process.
UPPER CONTROL LIMIT: Is the horizontal line on the control chart which show the range of variation, any point that fall above it be accepted by the manufacturer.
LOWER CONTROL LIMIT: Is another control limit, which show the range of variation in control chart that any sample point fall below it will not be accepted by the consumer.
STATISTICAL PROCESS CONTROL: Refers to the application of the method of statistical quality control to the monitoring of process.
1.3 AIMS AND OBJECTIVES OF THE STUDY
The aim of this research project is to apply the control charts (X-bar and R-bar chart) on the selected parameters and see how it affects the quality production of Cement Company of Northern Nigeria Plc, Sokoto.
1. To test whether samples meet the criteria associated with samples drawn from the same universe.
2. To find out whether that data are statistically uniform or some certain variation exist.
3. To find out whether the process is in control or out of control
1.4 SCOPE AND LIMITATION OF THE STUDY
There are factors that influenced the scope of this research project. In the first place the fact that this study was carried out on only the finished products of the cement company of northern Nigerian plc.
Limitations to the used parameters such as weight, record, water due to time constraint, problem of logistics and restriction to some parameters by the management. Therefore application of quality control charts would be restricted to X-bar and R-bar chart only.
1.5 SIGNIFICANCE OF THE STUDY
This work has been analyzed in terms of it work on the side of the organization concerned (quality control of business company) at the end of the analysis CCNN would be able to provide accurate information about the effect of control or out of control and also entails how to control business company.
1.6 DATA USED FOR THE STUDY
The data used for this research project is a secondary data specifically collected from the department of quality of the cement company of northern plc. Sokoto samples of 5 month consecutive reading were obtained and 20 samples are drawn at random from the production system for all the four parameters considered. The data is based on 2010 production for a period of months (June 2010-October2010).
CHAPTER TWO
2.0 LITERATURE REVIEW
2.1 INFORMATION ON CONTROL CHART
The control chart was invented by Walter A. Shewhart while working for Bell Lab in the 1920's. The company’s engineers had been seeking to improve the reliability of their telephony transmission system. Because amplifiers and other equipment had to be burrier underground there was business need to reduce the frequency of failure and repair by 1920 the engineers had already realized the important of reading variation in a manufacturing process. Moreover, they had realized the continual process adjustment in reaction to non-conformance actually increased variation and degraded quality. Shewahart framed the problem in terms of common and special causes of variation and on May 1924, wrote an intern memo introducing the control chart as a tool for disgusting between the two Dr. Shewhart's boss, Goege Edwarts, recalled "Dr. Shewhart prepared a little memorandum only about a page in length. About a third of that page was given over a simple diagram which we would all recognize today as a schematic control chart. The diagram and sort text which proceed and followed it, set forth all the essential principles and considerations which are involved in what we know today as process quality control. Shewhart stressed that bringing a production process into a state of statistical control, where there is only common cause variation and keeping it in control is necessary to predict future output and to manage a process economically Shewhart created the basic for the control chart and the concept of a state of statistical control by carefully designed experiments, while Dr. shewhart drew from pure mathematical statistical theories, he understood that data from physical processes seldom produces a "normal distribution curve" ) a Gaussian distribution, also commonly referred to as "bell curve") He discovered that observed variation in manufacturing data did not always behave the same way as data in nature. Dr. Shewhart concluded that while every process display variation, some process (common causes of variation), while others display controlled variation that is not present in the process causal system at all times (special causes of variation)
Moreover, Shewhart argued that, as processes subject to a special cause variation were inherently unpredictable the usual techniques of probability could not be used to separate special cause from common cause variation. He developed the control chart as statistical heuristic to distinguish the two types of variation. Both Deming and Shewhart advocate the chart as a means of assessing a process's state of statistical control and as foundation for forecasting. According to Elhence, et al (2007) chance variations are cumulative effects of a multiplication of minor cause which operate in a random fashion. These causes cannot be identified and be natural to any production process and the variations due to such factors are called "chance variation" or "natural variation" the causes operate in all variations is zero and error variance like standard deviation is constant it is for these reasons that their effect is called stable pattern of variation". This constant causes system is inherent in all production process and its effect cannot be eliminated in all production process and its effect cannot be eliminated.
Furthermore, as against chance variation the cause of which cannot be identified and effect of which cannot be eliminated, the assignable variation is due to such factors which can be identified and whose effects can be eliminated. These causes are not random such variations are often called 'chaotic' or ' erratic' or 'preventive variation'. These causes can be in any stage of the final production. These variations haves to be identified and their cause determined and eliminated before the production process is allowed to continue. These causes could be on account of defective raw materials, defective machine, defective handling of good machine.
2.2 COMMON TYPES OF CHART
The type of chart are often classified according to the type of quality characteristics that they are supposed to monitor, there are quality control charts from attribute specifically, the following charts are commonly constructed for controlling variables.
X-bar chart: in this chart the sample means are plotted in order to control the mean value of variable (e.g. size of piston ring, strength of materials, etc.) R-chart: in this chart the sample range are plotted in order to control the variability of
S-chart: in this chart sample standard deviations are plotted in order to control the variability of variable.
S**2 chart: in this chart the sample variances are plotted in order to control the variability of a variance.
In this research we are only using X-chart and R-chart.
2.3 HISTORICAL BACKGROUND OF CEMENT COMPANY NORTHERN NIGERIAN PLC, SOKOTO:
Cement company of northern Nigeria plc. (CCNN) was founded by the premier of the northern Region Alhaji Sir Ahmad Bello, and Sardauna of Sokoto it was incorporated in 1962 and commenced of 100,000 tons per annum, using the process to meet increasing demand for cement necessitated the expansion of the kalambaina production plant by commission of a second line with an installed capacity of 500,000 tons per annum in 1985, by the then head of state Major General Mohammadu Buhari. Thereafter, in 1986 the first line was shut down due to uneconomic mode of operation, thus learning the plant with a rated output of 500,000 tons per annum. Under the privatization and commercialization program of the Gen Babangida Administration in 1992. The federal government disinvested about 20% of its holding in the company and sold it in Nigerian public.
Furthermore, under the civilian administration of Chief Olusegun Obasanjo, cement company of northern Nigeria plc. Was embarked a one of the companies to be fully privatized in July 2000. Therefore public binding for the company was concluded and international A.S.N of Norway member of Heidelberg cement group was appointed as core investors and technical partner of the company.
2.4 METHOD OF QUALITY CONTROL IN CCNN PLC, SOKOTO
The method of data collection adopted by the company is random sampling. In which every member of the production has an equal and independent chance of selection. This involve taken a sample of product into a laboratory for analysis. While in the laboratory the sample undergo various form of analysis which include; Heart on ignition, water consumption, expansion initial setting, final setting, Blaine and insoluble residue. The values of these parameters are in ranges, if found to be drifting out of range (either below or above), the quality control unit will inform the production unit for appropriate corrective action.
2.4.1TERMILOGY USED IN METHOD OF QUALITY CONTROL IN CCNN PLC, SOKOTO
INCOMING RAW MATERIAL CONTROL
Cement constitutes of line, Gybsum, clinker and some assorted chemicals do not undergo much security because they have passed checks before importation.
IN PROCESS CONTROL
This involves making sure that all ratios are in correct proportions. The wet processes is carefully controlled and mentored to ensure that correct temperature and pressure are maintained as required.
FINISHED PRODUCT
This involves taken a sample of the product to laboratory for analysis to ensure compliance with quality standard in all parameters. Once the raw materials and in process have been ascertained, the bulk of quality control process fall on the finished products and high frequency checking half hour.
2.5 CONTROL CHART FOR X-BAR CHART
The control chart is primarily designed to control the variation in the process average. The following steps are involved in the preparation of X-chart
1. Taking the measurement sample units which should be selected at random from process product. Measurement must be done very carefully be experts with the help of sophisticated equipment’s.
2. Selecting the number subgroup at random so that each unit in the lot has the same chance of being selected. The samples should not contain less than reasonable number of items.
3. Making the following calculations.
Mean of various samples X1, X2,...,Xn are taken
a) The mean of sample is given by
Xi = i=1,2,3,..,k j=1,2,3,..,k (2.1)
b) The mean of all sample mean is given by
Xi= i=1,2,3,..,k j=1,2,3,..,k (2.2)
c) Range of a sample is given by
Ri= Xij max - Xij min i = 1,2,3,..,k j = 1,2,3,..,k (2.3)
d) The mean of various sample range
Ri= for i=1, 2, 3, .,k
4. Setting the control limits since we do not know the values of µ and then we used best possible estimate of 3. The mean of the sample mean or X represent µ (the mean of the universe) and R/d2 represents the standard deviation. d2 is a constant depending on size.
Thus the control limits are given by
X ± 3 or
X ± 3 R (2.5)
But, A2 = (2.6)
̤̇ X + A2 R = UCL and (2.7)
X - A2 R = LCL (2.8)
Where n=number of observations
k=number of all samples
UCL=Upper control limit
LCL= Lower control limit
A2 and d2 are values from 3 table
5. Constructing the X-bar chart is by taking the sample number on the horizontal scale (X-bar axis) and the quality level on the vertical scale (Y-axis). Sample point (means of sample X1, X2, ... Xn) may be plotted by dot and the central line or X-bar chart line prominently. The UCL and LCL may also be drawn by dots or line.
Interpreting the control chart will be if all the plotted points are within the tolerance limits, then the process is in control if some points are outside the tolerance limit (between UCL and LCL ) the process should be stopped until the cause is rectified.
2.6 CONTROL CHART R-CHART (RANGE)
The range chart or R-chart is constructed by controlling the variations in the dispersion or variability of the products: Generally, R-chart is used simultaneously so that the variability in means and dispersion are studied at the same time. This type of analysis gives a better idea about whether the process is in control or not. The procedure for constructing the R-chart, this is most the same as that for X-bar chart.
The steps for constructing R-chart are as follows:
1. Calculating the range of each sample (R) and the mean of all the sample range (R) which is already calculated.
2. Calculating the UCL and LCL since and are unknown.
UCL= D4R
LCL=D3R
Where D3 and D4 are values from 3 table
3. R-chart is constructed in the same way as that of X-bar chart
4. Interpretation is also the same way as that of X-bar chart.
3.0 CHAPTER THREE: DATA ANALYSIS
3.1 INTRODUCTION
This chapter is purposely designed to analyze the following parameters; (weight, Water consumption, Expansion and Blaine record respectively.) on the reduction of cement in Sokoto Cement Company of Northern Nigeria plc, using SPSS software.
Weight Record
X
X
UCL
LCL
52.00
51.00
49.00
52
51.00
50.00
50.2293
51.6683
48.6971
51.00
52.00
50.25
51
52.00
51.25
50.2293
51.6683
48.6971
49.00
51.25
50.00
49.25
48.00
49.05
50.2293
51.6683
48.6971
50.40
52.04
50.00
48.45
50.00
50.25
50.2293
51.6683
48.6971
51.00
51.00
51.00
51.35
48.00
50.47
50.2293
51.6683
48.6971
51.00
49.00
49.25
50
51.00
50.04
50.2293
51.6683
48.6971
52.25
48.00
50.00
49
51.00
49.94
50.2293
51.6683
48.6971
52.45
50.25
48.00
51.25
49.45
50.68
50.2293
51.6683
48.6971
50.00
50.00
48.25
49.45
49.45
49.38
50.2293
51.6683
48.6971
48.05
50.00
49.00
50
50.00
49.35
50.2293
51.6683
48.6971
48.49
50.00
51.00
51.35
50.00
49.77
50.2293
51.6683
48.6971
52.00
50.00
50.00
50.00
50.00
50.06
50.2293
51.6683
48.6971
50.00
50.00
51.00
50.00
49.00
49.08
50.2293
51.6683
48.6971
51.00
50.00
52.00
52.00
50.00
50.08
50.2293
51.6683
48.6971
52.25
49.00
51.00
51.00
51.00
51.05
50.2293
51.6683
48.6971
51.00
50.00
50.00
50.00
51.00
50.06
50.2293
51.6683
48.6971
50.00
51.00
50.00
48.54
50.00
49.89
50.2293
51.6683
48.6971
50.00
49.25
49.00
51.00
50.00
49.09
50.2293
51.6683
48.6971
48.40
50.00
51.00
51.00
49.00
49.89
50.2293
51.6683
48.6971
49.00
51.00
49.00
49.45
49.45
49.99
50.2293
51.6683
48.6971
FIG 3.1:X-bar CHART FOR WEIGHT RECORD USING SPSS.
THE ABOVE SHOWS THAT THE WEIGHT RECORD PER BAG IS UNDER CONTROL WITH NORMAL BEHAVIOUR SINCE MEAN= 50.1827, ULC= 51.6683, LCL=48.6971 FOR X-bar CHART.
Weight Record
Ri
–
R
UCL
LCL
52
51
49
52
51
3.00
2.5755
6.4027
0
51
52
50.25
51
52
2.75
2.5755
6.4027
0
49
51.25
50
49.25
48
3.25
2.5755
6.4027
0
50.40
52.04
50
48.45
50
3.95
2.5755
6.4027
0
51
51
51
51.35
48
3.35
2.5755
6.4027
0
51
49
49.25
50
51
2.00
2.5755
6.4027
0
52.25
48
50
49
51
4.45
2.5755
6.4027
0
52.45
50.25
48
51.25
49.45
3.00
2.5755
6.4027
0
50
50
48.25
49.45
49.45
2.00
2.5755
6.4027
0
48.05
50
49
50
50
1.75
2.5755
6.4027
0
48.49
50
51
51.35
50
2.86
2.5755
6.4027
0
52
50
50
50
50
2.00
2.5755
6.4027
0
50
50
51
50
49
1.00
2.5755
6.4027
0
51
50
52
52
50
2.00
2.5755
6.4027
0
52.25
49
51
51
51
3.25
2.5755
6.4027
0
51
50
50
50
51
1.00
2.5755
6.4027
0
50
51
50
48.54
50
2.55
2.5755
6.4027
0
50
49.25
49
51
50
1.91
2.5755
6.4027
0
48.40
50
51
51
49
2.58
2.5755
6.4027
0
49
51
49
49.45
49.45
1.95
2.5755
6.4027
0
FIG 3.1: R-bar CHART FOR WEIGHT RECORD USING SPSS.
THIS SHOWS THE RANGE OF WEIGHT RECORD WHERE THE RANGE= 2.5755, UCL=6.4027, LCL=0
Water Consumption
X
X
UCL
LCL
132
132
132
134
134
132.8
131.3900
134.2164
128.5636
128
128
130
132
130
129.6
131.3900
134.2164
128.5636
130
132
132
135
130
131.8
131.3900
134.2164
128.5636
128
130
130
134
134
131.2
131.3900
134.2164
128.5636
130
130
128
134
134
131.2
131.3900
134.2164
128.5636
132
123
130
134
132
130.2
131.3900
134.2164
128.5636
130
130
130
134
132
131.2
131.3900
134.2164
128.5636
128
130
128
134
134
130.8
131.3900
134.2164
128.5636
130
130
134
136
134
132.8
131.3900
134.2164
128.5636
128
134
130
134
134
132
131.3900
134.2164
128.5636
130
130
132
134
134
132
131.3900
134.2164
128.5636
132
132
130
132
132
131.6
131.3900
134.2164
128.5636
130
130
132
133
134
131.8
131.3900
134.2164
128.5636
130
130
130
130
134
131.8
131.3900
134.2164
128.5636
130
130
132
128
134
130.8
131.3900
134.2164
128.5636
130
130
132
128
134
130.8
131.3900
134.2164
128.5636
130
128
130
128
132
129.6
131.3900
134.2164
128.5636
130
130
132
128
134
130.8
131.3900
134.2164
128.5636
131
132
132
135
132
132.4
131.3900
134.2164
128.5636
130
130
134
134
130
131.6
131.3900
134.2164
128.5636
131
132
132
135
134
132.8
131.3900
134.2164
128.5636
Fig 3.2: X-bar CHART FOR WATER CONSUMPTION USING SPSS.
THE ABOVE SHOWS THAT THE WATER CONSUMPTION IS UNDER CONTROL SINCE MEAN=131.3900, UCL=134.2164, LCL=128.5636
Water Consumption
Ri
R
UCL
LCL
132
132
132
134
134
2
4.700
11.6812
0.00
128
128
130
132
130
4
4.700
11.6812
0.00
130
132
132
135
130
5
4.700
11.6812
0.00
128
130
130
134
134
6
4.700
11.6812
0.00
130
130
128
134
134
6
4.700
11.6812
0.00
132
123
130
134
132
11
4.700
11.6812
0.00
130
130
130
134
132
4
4.700
11.6812
0.00
128
130
128
134
134
6
4.700
11.6812
0.00
130
130
134
136
134
8
4.700
11.6812
0.00
128
134
130
134
134
6
4.700
11.6812
0.00
130
130
132
134
134
4
4.700
11.6812
0.00
132
132
130
132
132
0
4.700
11.6812
0.00
130
130
132
133
134
4
4.700
11.6812
0.00
130
130
130
130
134
4
4.700
11.6812
0.00
130
130
132
128
134
6
4.700
11.6812
0.00
130
128
130
128
132
2
4.700
11.6812
0.00
130
130
132
128
134
2.8
4.700
11.6812
0.00
131
132
132
135
132
4
4.700
11.6812
0.00
130
130
134
134
130
4
4.700
11.6812
0.00
131
132
132
135
134
4
4.700
11.6812
0.00
FIG 3.2: R-bar CHART FOR WATER CONSUMPTION USING SPSS.
THE ABOVE SHOWS R-CHART OF WATER CONSUMPTION WITH MEAN=4.700, ULC= 11.6812, LCL= 0.0000
Expansion Record
X
X
UCL
LCL
0.5
0.5
1
0.5
1
0.7
0.8250
1.1903
0.4597
0.5
1
0.5
1
1
0.8
0.8250
1.1903
0.4597
1
0.5
1
1
1
0.9
0.8250
1.1903
0.4597
1
1
1
0.5
0.5
0.8
0.8250
1.1903
0.4597
1
1
1
1
0.5
0.9
0.8250
1.1903
0.4597
0.5
1
1
0.5
0.5
0.7
0.8250
1.1903
0.4597
1
0.5
0.5
1
1
0.8
0.8250
1.1903
0.4597
0.5
1
1
1
1
0.9
0.8250
1.1903
0.4597
1
1
1
0.5
0.5
0.8
0.8250
1.1903
0.4597
1
0.5
0.5
1
1
0.8
0.8250
1.1903
0.4597
0.5
1
1
1
1
0.9
0.8250
1.1903
0.4597
1
0.5
1
1
1
0.9
0.8250
1.1903
0.4597
1
1
0.5
0.5
0.5
0.7
0.8250
1.1903
0.4597
1
0.5
1
1
1
0.9
0.8250
1.1903
0.4597
0.5
1
1
1
1
0.9
0.8250
1.1903
0.4597
1
1
0.5
0.5
0.5
0.7
0.8250
1.1903
0.4597
1
1
1
1
1
0
0.8250
1.1903
0.4597
1
1
1
0.5
0.5
0.9
0.8250
1.1903
0.4597
0.5
1
1
1
1
0.9
0.8250
1.1903
0.4597
1
0.5
1
0.5
0.5
0.8
0.8250
1.1903
0.4597
FIG 3.3 Expansion record of sample of cement factory
FIG 3.3: X-bar CHART FOE EXPANSION RECOR USING SPSS.
THE ABOVE CHART IS THE X-bar CHART OR EXPANSION RECOD AND THE CHART IS UNDER CONTROL WITH THE NEED FOR IMPROVEMENT WITH THE MEAN= 0.8250, UCL= 1.1903, LCL= 0.4597
Expansion Record
Ri
R
UCL
LCL
0.5
0.5
1
0.5
1
0.5
0.475
1.1808
0.00
0.5
1
0.5
1
1
0.5
0.475
1.1808
0.00
1
0.5
1
1
1
0.5
0.475
1.1808
0.00
1
1
1
0.5
0.5
0.5
0.475
1.1808
0.00
1
1
1
1
0.5
0.5
0.475
1.1808
0.00
0.5
1
1
0.5
0.5
0.5
0.475
1.1808
0.00
1
0.5
0.5
1
1
0.5
0.475
1.1808
0.00
0.5
1
1
1
1
0.5
0.475
1.1808
0.00
1
1
1
0.5
0.5
0.5
0.475
1.1808
0.00
1
0.5
0.5
1
1
0.5
0.475
1.1808
0.00
0.5
1
1
1
1
0.5
0.475
1.1808
0.00
1
0.5
1
1
1
0.5
0.475
1.1808
0.00
1
1
0.5
0.5
0.5
0.5
0.475
1.1808
0.00
1
0.5
1
1
1
0.5
0.475
1.1808
0.00
0.5
1
1
1
1
0.5
0.475
1.1808
0.00
1
1
0.5
0.5
0.5
0.5
0.475
1.1808
0.00
1
1
1
1
1
0.5
0.475
1.1808
0.00
1
1
1
0.5
0.5
0.5
0.475
1.1808
0.00
0.5
1
1
1
1
0.5
0.475
1.1808
0.00
1
0.5
1
0.5
0.5
0.5
0.475
1.1808
0.00
FIG 3.3 expansion record of sample of cement factory
FIG 3.3: R-bar CHART FOR EXPANSION RECORD USING SPSS.
THE ABOVE SHOWS THE R-bar CHART OF EXPANSION RECORD IT INDICATES THAT IS UNDER CONTROL AND ALSO NEED IMPROVEMENT WITH MEAN= 0.4750, UCL=1.1808 , LCL=0.0000
Blaine Record
X
X
UCL
LCL
3960
3980
4220
3930
3960
4010
3954.2000
4183.0050
3725.3950
3840
4020
4170
4030
4020
4016
3954.2000
4183.0050
3725.3950
3960
4130
4240
3970
3840
4020
3954.2000
4183.0050
3725.3950
3910
4050
4090
3940
4040
4006
3954.2000
4183.0050
3725.3950
3840
4020
4230
3960
4070
4018
3954.2000
4183.0050
3725.3950
3840
3970
4070
4020
3960
3972
3954.2000
4183.0050
3725.3950
3840
3970
3970
3930
3850
3912
3954.2000
4183.0050
3725.3950
3820
4020
3960
3950
3940
3946
3954.2000
4183.0050
3725.3950
3910
3960
3940
4300
3890
3982
3954.2000
4183.0050
3725.3950
3920
4190
3970
3970
3830
3974
3954.2000
4183.0050
3725.3950
3840
4240
3970
3890
3830
3970
3954.2000
4183.0050
3725.3950
3920
4020
4030
3920
3870
3936
3954.2000
4183.0050
3725.3950
3780
4240
3970
3890
3930
3990
3954.2000
4183.0050
3725.3950
3820
4230
4030
3970
3870
3976
3954.2000
4183.0050
3725.3950
3890
3840
4140
3880
3950
3926
3954.2000
4183.0050
3725.3950
3840
4010
4040
3850
4040
3946
3954.2000
4183.0050
3725.3950
3810
3950
3810
3760
3980
3862
3954.2000
4183.0050
3725.3950
3910
4080
3920
3880
3890
3942
3954.2000
4183.0050
3725.3950
3810
3950
3810
3760
3980
3862
3954.2000
4183.0050
3725.3950
3870
3950
3960
3940
4020
3945
3954.2000
4183.0050
3725.3950
FIG 3.4 X-bar CHART OF BLAINE RECORD USING SPSS.
THE ABOVE RESULTSHOWS THAT THE BLAINE ARE UNDER CONTROL WITH NORMAL BEHAVIOUR WITH MEAN=3954.2000, UCL=4183.0050, LCL=3725.3950
Blaine Record
Ri
R
UCL
LCL
3960
3980
4220
3930
3960
290
267.5
702.2947
0.00
3840
4020
4170
4030
4020
180
267.5
702.2947
0.00
3960
4130
4240
3970
3840
400
267.5
702.2947
0.00
3910
4050
4090
3940
4040
430
267.5
702.2947
0.00
3840
4020
4230
3960
4070
220
267.5
702.2947
0.00
3840
3970
4070
4020
3960
230
267.5
702.2947
0.00
3840
3970
3970
3930
3850
130
267.5
702.2947
0.00
3820
4020
3960
3950
3940
160
267.5
702.2947
0.00
3910
3960
3940
4300
3890
480
267.5
702.2947
0.00
3920
4190
3970
3970
3830
360
267.5
702.2947
0.00
3840
4240
3970
3890
3830
410
267.5
702.2947
0.00
3920
4020
4030
3920
3870
190
267.5
702.2947
0.00
3780
4240
3970
3890
3930
350
267.5
702.2947
0.00
3820
4230
4030
3970
3870
160
267.5
702.2947
0.00
3890
3840
4140
3880
3950
320
267.5
702.2947
0.00
3840
4010
4040
3850
4040
250
267.5
702.2947
0.00
3810
3940
3810
3560
3960
220
267.5
702.2947
0.00
3940
4080
3920
3880
3890
200
267.5
702.2947
0.00
3710
3840
3810
3520
3860
220
267.5
702.2947
0.00
3870
3950
3960
3940
4020
150
267.5
702.2947
0.00
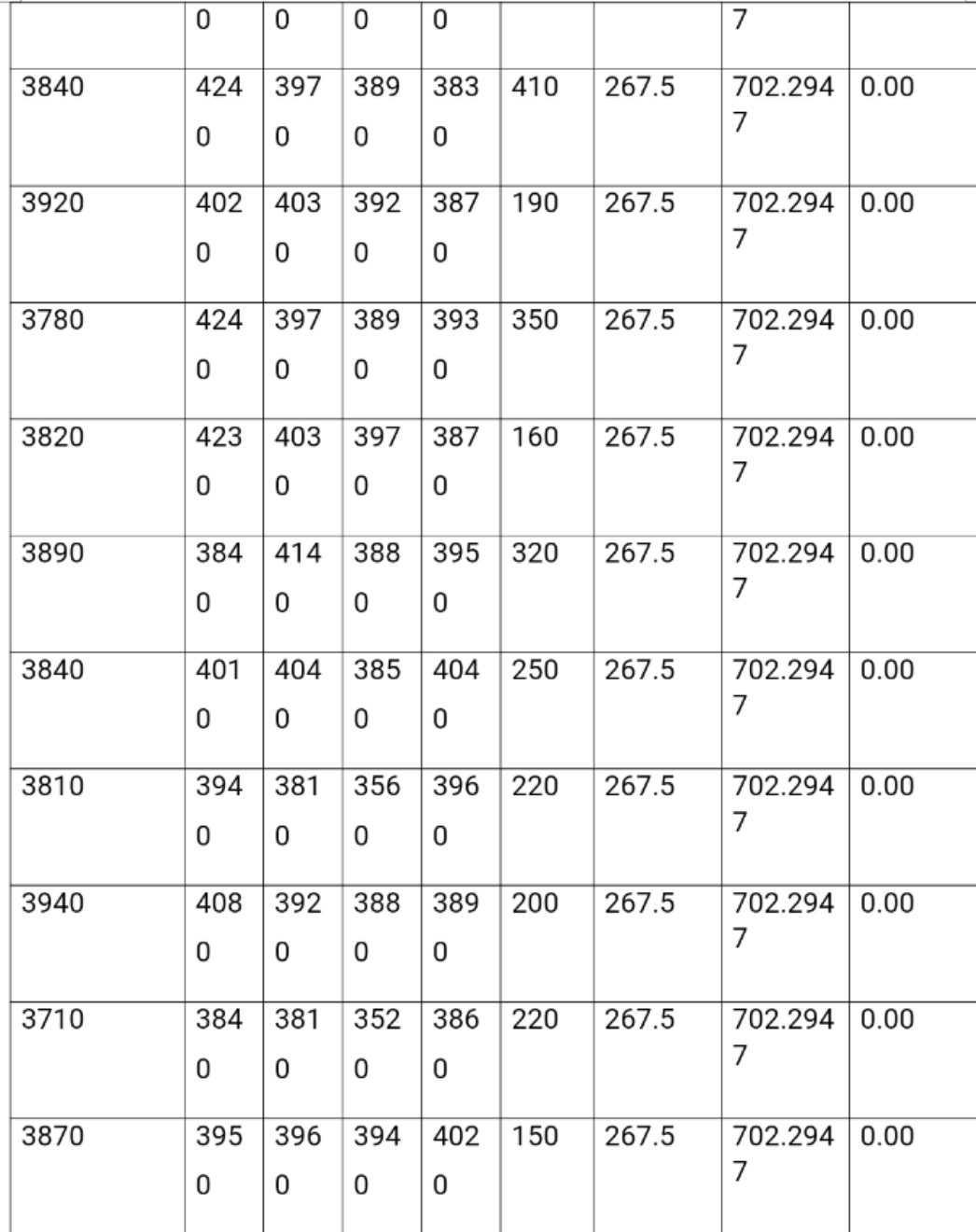
FIG 3.4 R-bar CHART FOR BLAINE RECORD SPSS.
THE ABOVE SHOWS THE BLAINE RECORD OF R-CHART WITH MEAN= 282.5000, UCL=702.2947, LCL= 0.0000
CHAPTER FOUR
4.0 SUMMARY
In summary ,chapter one deals with the with the general introduction of quality, the statement of problems, aims and objective of the study .It also looks at the Definition of some basic concept of quality control, scope and limitation and
Also method of data collection.
In chapter two I looked at the literature review of control chats, the causes of variation (chance and assignable cause of variation) method of quality control in CCNN plc, sokoto. Likewise control of X-bar chat and R-chat and their computation were discussed, including the derivation of upper and lower control limit.
Chapter three deals with data analysis based on the selected parameters which includes; weight, water consumption, expansion and Blaine record, using SPSS .Interpretation was also made based on our findings.
Finally chapter four deals with summary of the research work, valuable Conclusion and recommendation were made.
4.1 CONLUSION:
Finally, based on the analysis obtained in chapter three, we can conclude that that the production process of Cement Company of northern Nigeria plc is under control, with normal behavior in weight and Blaine record. But expansion and water consumption record shows there is need for improvement since one approaches the lower control of R –chart.
It is our believe that this stage research project the objectives have been achieved and the significant appreciated. Looking back at the application it will be seen that the control chart has always served as reliable way of analyzing, organizing and appreciating production system .A control chart give every indication 0f continuing to be a powerful tool for the generation of standard product. Indeed the study control chart is rewarding.
4.2 RECOMMENDATION:
The following are recommended to cement company of northern Nigeria plc sokoto.
The study shall be dully restudied by the company and it should be implemented without further delay if satisfied with every bit of the research
The method control charts should be documented on a manual to serve a quality control unit.
The company should try as much as possible to improve all other department so as to improve quality of its services.
The new method should be properly maintained to enhance efficient and standard production system.






0 Response to "control charts (X-bar and R-bar chart) on the selected parameters and see how it affects the quality production of Cement Company of Northern Nigeria Plc, Sokoto"
Post a Comment